The general connection between wavelength L, pitch F, and sound speed c is

  F = c / L

The pitch of an organ pipe is controlled by its resonator, often a tube of constant circular or rectangular cross section. The length of this tube is in principle either a half wavelength (open flue pipes) or a quarter wavelength (stoppered flue pipes, reed pipes). Further refinements apply to this rule. One is the 'end correction', the physical length of the tube has to be made somewhat shorter because the air immediately outside its open end(s) takes part in the resonant motion. Another is when the tube flares some way, giving modified ratios between wavelength and resonator length.

A pipe is tuned by adjusting its length at some specific air temperature. The problem addressed here is in a short time perspective, based on theory, namely how the pitch of the pipe will then vary at other temperatures as consequence of the change in sound speed.

There are additional tuning issues, practical ones beyond the present scope. One is that humidity in particular may influence the air supply blowing pressure, e.g. by effects from seizing actuators and leakage. Such blowing variation may cause changes in pipe pitch and tonal onset. Another is that the pipe length adjustment may be upset over time from thermal and humidity variations, or mechanical vibrations. With many pipe designs the tuning element (e.g. tuning slide, sleeve, stopper, or wire) may move cumulatively from the 'ratchet effect'. This means that very small shape distortions, though individually harmless, are not perfectly cancelled when their causing disturbance ends. Instead they may add up such that the pipe goes out of tune in the long run.

Speed of sound in air vs. temperature and humidity

The basic expression for the speed of sound c in meter/second in a gas is

If pressure is changed at constant temperature, then density will vary precisely in the same proportion. This leaves c unaffected, sound speed is independent of barometric pressure.

But contrarily, at constant pressure, density will vary inversely with absolute temperature. This renders an additional, commonly cited expression, for air

showing how speed at 0 deg. centigrade is modified at absolute temperature T_K (in Kelvin) or T_C (in centigrade).

I never saw published figures on sound speed variation with humidity. But in references [1] and [2] I could allocate raw data on density, adiabatic constants, and water vapor saturation pressures. The saturation pressure is strongly dependent of temperature and indicates the relative influence of the water vapor.  Following a somewhat tedious proportioning sequence of computations in an Excel sheet, I finally obtained figures for sound speed in meters/second, for dry and for water saturated air:
 

For practical tuning purposes these figures could be converted into relative pitch in cents, 100 cents = one semitone. The formula

tells how many cents pitch will change at sound speed c_x, compared to pitch at some reference speed c₀. (An octave is a factor of 2,  containing 12 semitones, 100 cents each, making for 1200 cents). Arbitrarily selecting dry air at 20 deg. centigrade for reference, the result is graphically shown in this diagram:

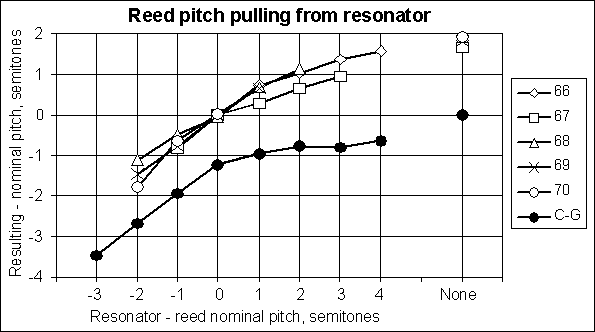
Humidity does have some effect on sound speed, but small enough to be practically negligible in most cases.

Thermal expansion of materials

Also the physical size of a pipe varies with temperature. For tin, lead, and zinc the elongation with temperature is in the range 2 to 3*10-5 (20-30 ppm) per degree centigrade. 1 cent being a frequency change of 578 ppm, you would need something like +20 degrees temperature change to invoke -1 cent detuning due to thermal expansion. Some 60 times smaller effect than from the corresponding sound speed change in air, and with opposite sign. For practical purposes this effect can be neglected.

Hygroscopic swelling of wood

Wood is a 'live' material that swells and shrinks depending on humidity, with a variation between species around 1:2. From fresh cut 'green' having about 60% moist, to usable dried state, shrinkage is little along the grain, typically. 0.1%, but considerable across, 10% tangentially, 5% radially, ref [3]. After drying this dimensional variation is less than half of these figures. Then the wood 'equilibrium moisture content' is between typically 0 and 30 % as ambient relative humidity goes from 0 to 100%, with a time constant from days to months.

As numeric example we may assume no length variation, but a 1% variation in diameter of a pipe. That would mean a corresponding change in the end correction and thus perhaps one tenth of this in total effective pipe length. But 0.1% corresponds to 1.7 cents, a rather small direct effect on the tuning. The more important effect from hygroscopic swelling and shrinkage would be its cumulative actions on the position of any tuning sliders, etc.

If a wooden pipe has cracks in it, then humidity variations may cause large and unpredictable changes, ranging from detuning to silencing the pipe altogether.

Reed pipes

The stiffness, density, etc. of materials in the reed mechanism are held to be insensitive to temperature, very small effects anyway. This is often misinterpreted such that reed pipe tuning would be insensitive to temperature. But the pipe fundamental is determined by reed and air column in combination. The coupling between these varies with the particular pipe design. The detuning vs. temperature in reed pipes may be anything between 0 and 100% of that of flue pipes.

For illustration, here follows a comprehensive graph of my measurements of reed pitch pulling by a cylindrical quarter wave resonator. The horizontal axis is an imposed variation of the resonator tuning, away from its normal frequency, in semitones. The vertical axis is the resulting pitch in semitones deviation from nominal.

1. Beating reeds (open symbols). Objects are a set of five pipes from my clarinet rank, f#-a#, MIDI 66-70, 370-466 Hz. These were selected because they happen to be built with equal resonator diameters, 25 mm. Consequently I could swap resonators between them without touching the sensitive reeds in any way, throughout measurements the reeds were not even taken out of their pipe boots. - These pipes are designed to work with a resonator. Their nominal pitch is closely matched when their native resonators are mounted (at horizontal axis zero mark). With the same reed, switching to other resonators (i.e. in steps of semitones, 100 cents), pitch will change partly in the direction of resonator frequency. When no resonator is installed (mark None) the reeds take a pitch 1.7 - 2 semitones higher than nominal.

2. Free reeds (filled circles). A set of eight harmonium reeds, C-G, MIDI 36-43, 65-98 Hz, were run in one specific resonator 46 mm dia., 1.03 m length, tuned to E, MIDI 40, 82 Hz. - These reeds are designed to work without a resonator, and doing so their nominal pitch is closely matched (mark None). When adding the resonator pitch is always lowered, also when the resonator is tuned higher than the reed. E.g. at mark 0 where reed and resonator have individually the same tuning, then the resulting pitch is 1.2 semitones (120 cents) lower.

These curves do not extend leftwards; with resonators tuned still lower the coupling between reed and resonator is lost. The beating reeds then 'crack', the free reeds return toward their natural pitch.

The general slope is about the same, both for beating and free reeds, approximately 0.7. This may well vary, depending on resonator shape, or on its diameter in relation to reed size.

Conclusions

The main rule is: In dry air the pitch of flue pipes will rise with temperature 3 cents per centigrade, 1.7 cents per deg F. Equivalently, a quarter semitone (25 cents) per 8 degrees centigrade or per 15 deg F.

In saturated humid air (100 % RH) the effect is some 13% larger (about 3.4 ct/deg C), marginally worthwhile to account for.

Pipe detuning due to thermal or hygroscopic expansion of the pipe bodies is negligible by comparison.

Contrary to common saying also reed pipes are affected similarly, but to a lower degree dependent on design, typically maybe 70%. Though the reed itself is negligibly affected by temperature the resonator is so, and this will pull the resulting pitch.

References

[1]  Kinsler, Frey & al: Fundamentals of acoustics. New York, Wiley 1950, 1962, 1982. ISBN 0-471-09410-2.
[2]  'Hütte', des Ingenieurs Taschenbuch. Berlin, Ernst & Sohn 1911.
[3]  Eckelman C A: The Shrinking and Swelling of Wood and Its Effect on Furniture,  http://www.fnr.purdue.edu/PubsOnLine/fnr163.pdf