| You Are Not Logged In | Login/Get New Account |
|
Please Log In. Accounts are free!
Logged In users are granted additional features including a more current version of the Archives and a simplified process for submitting articles. |
|
MMD
 Tech
Tech
 leakmeter
leakmeter
|
|
Leak Meter e-mail: johan@speech.kth.se What is flow resistance? You may be familiar with Ohm's law in electricity. When you put a voltage U (Volts) across a resistor, there will flow a current I (Amperes) through it. The ratio between these define the resistance (in Ohms) R=U/I. In hydromechanics and acoustics you make a classical analogy: Instead of voltage U you use driving pressure P (Pascals=N/m^2). Instead of current I you use volume flow Q (m^3/sec). And you define resistance as R=P/Q, driving pressure P divided by flow Q. Thus acoustic resistance is measured in the SI unit (N/m^2)/(m^3/s)=Ns/m^5. If you like better to measure pressure in inches of water column and flow in for instance cubic inches per second, that works just fine. The resistance will then be in the cryptic unit of 'water seconds per square inch', and the number will be of course be different from the SI rating. What for? The focus here is to find out the flow loss from a leaky container. For instance how much air will leak through the wood in a valve block or through the cloth in a bellows. If you feed it with the pressure P, then the flow will be Q=P/R. There are several other applications relevant to organ technology, for instance to find the flow through a bleed hole, to find out what diameter you need for a duct in the supply system, or what diameter you need for a tube between valve and tracker bar. There may be reason to come back to these issues later.
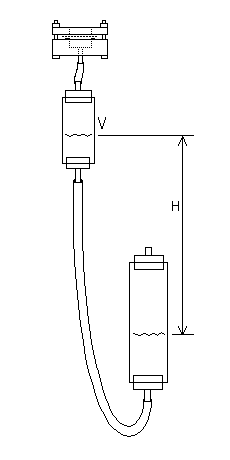
The meter consists of two vessels joined by a flexible tube, half filled with water. The upper, and smaller vessel has volume V. Before measurement you lift the other vessel enough that this volume V is completely filled with water and you connect the sample to measure. To start measurement you lower the other vessel a known distance. Then you measure the time T it takes for the volume V to be emptied of water. The flow of air is then simply Q=V/T (m^3/s). During the measurement the sample is subject to the (under-) pressure P=H*g*rho (Pascals), where H (meters) is the average water column height, g=9.81 m/s^2 is the acceleration of gravity, and rho=1000 kg/m^3 is the density of water. I have fabricated the vessels from short pieces of transparent PVC tube, 1.5" i.d., a rubber plug each end with holes for short 1/4" brass tubes to match the connecting hose, some 4 ft of 1/4" i.d., see figure. For measurement on materials specimens (like wood slices, fabrics, leather) I connect a little cavity with a rubber gasket (cut from bicycle hose) and an overlay piece to press the specimen tightly against the gasket with four bolts. Removing the bolts and overlay piece the lower part of the adapter can also be clamped against bigger objects. Before use you must check two things: 1. The meter and specimen adapter must be airtight - put a metal plate as specimen and check that there is no flow. This criterion tells the highest resistance you can measure. Seal with glue or lacquer if necessary. 2. Establish the resistance of the meter itself. Leave the meter open and find the time it takes for the water to run out of V. This criterion suggests the lowest resistance you can measure. The value you get from this 'short circuit' test should be subtracted from your subsequent measurements on real specimens. The three things you know from the measurement is the volume V, the pressure indirectly from the column height H, and the time T. By manipulation of the definitions you arrive at the resistance of the sample R=P/Q=g*rho*H*T/V=9810*H*T/V (H in meters, T in seconds, V in cubic meters) This resistance measure is most useful on complete components in a system, like a valve, or a cavity with a pouch. For surface materials like leather, paper, or cloth it may be more useful to give a 'specific resistance' or 'area resistivity' Rs=R*As (Ns/m^3), where As is the area of the sample. To estimate the leak resistance of e.g. a complete bellows having a total surface Ab of the same material, divide to get R=Rs/Ab. ----------------------------------------------------------------------------- (Author JLs 990403) |
|
|
|
|
|
|
|
|
|
CONTACT FORM: Click HERE to write to the editor, or to post a message about Mechanical Musical Instruments to the MMD Unless otherwise noted, all opinions are those of the individual authors and may not represent those of the editors. Compilation copyright 1995-2025 by Jody Kravitz. Please read our Republication Policy before copying information from or creating links to this web site. Click HERE to contact the webmaster regarding problems with the website. |
|
|
||||||
|
 A
practical resistance meter for leak testing
A
practical resistance meter for leak testing